



Next: Two-component Hartree-Fock and DFT
Up: Miscellaneous
Previous: Totally symmetric singlets for
Contents
Index
Average of high-spin states:
n electrons in MO with
degenerate nir.
| a |
= 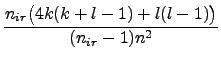 |
|
| b |
= 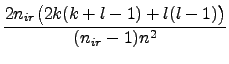 |
|
where:
k = max(0, n - nir) ,
l = n - 2k = 2S (spin)
This covers most of the cases given above. A CSF results only if
n = {1,(nir - 1), nir,
(nir + 1),
(2nir -1)} since there
is a single high-spin CSF in these cases.
The last equations for a and b can be rewritten in many ways, the probably most concise form is
| a |
= 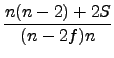 |
|
| b |
= 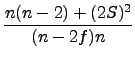 . . |
|
This applies to shells with one electron, one hole, the high-spin couplings of half-filled shells and those with one electron more ore less. For d2, d3, d7, and d8 it represents the (weighted) average of high-spin cases: 3F + 3P for d2,d8, 4F + 4P for d3, d7.




Next: Two-component Hartree-Fock and DFT
Up: Miscellaneous
Previous: Totally symmetric singlets for
Contents
Index
TURBOMOLE
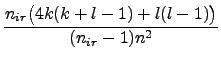
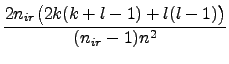
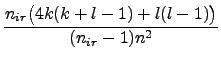
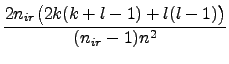
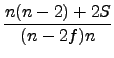
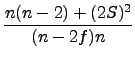 .
.